You’re going on a road trip with the kiddos. No tablets! No devices! That’s what they did in the good ol’ days. Ever wonder why the ol’ days are always good for every generation? Makes me what to believe that human existence is monotonically depressing in time. But at the same time, the future looks bright! Reconciling these two means that the now is miserable and we’re always at some type of (global) happiness minimum.
So this is Summer Excursion #6! If you are wondering where the other Summer Excursions are, just follow this summermath link.
As a pre-internet era kid, my parents engaged us in all sorts of math and word play when we went on road trips. But that was then, when times were simpler. This is now, when apparently times are more complex? So I give you three, device-free road trip math activities. Feel free to modify these to your liking, to your children’s math level, or to your geography (I’m assuming US geography, but I know I have plenty of readers from Germany and the UK)!
Activity #1, Are we there yet?
These works well when your journey takes you through moderate to highly populated areas. We need cars! One of the things we used to do as a kid was to find “cool” license plates. That meant spying custom plates or non-custom plates that seemed like they were saying something (like HLP-34R — “Help Ear”). These little scavenger hunts kept us entertained throughout those multi-hour car rides.
A math-y version of this works like this. Spy license plates and add the digits. Keeping spying until you’ve reached a sum of over 100. How many license plates did you need? What if you got to choose which license plates you wanted to use for your sum? If you have two kiddos in the car you can make this cooperative or competitive depending on how much screaming you want in the car (I encourage cooperation! Or if you want competition, parent(s) vs kid(s)). Can you get to a sum of over 100 in 10 or fewer license plates?
Activity #2, I’m thinking of a word …
Sometimes the supply of license plates is scant, making Activity #1 a chore and a bore. No problem! I have a solution for that. Either you can do this activity as an “I spy” type or have it be a bit more free form. Let’s see if you and I can do it even though we are currently in a writer-reader interaction. It goes something like this.
First, let’s see if you can figure out the rules. In the car, absent visual reading (as opposed to mental reading), this can be a bit more of a challenge, but I’ve done this with 2nd and 3rd graders and they are able to lock on pretty quickly without any visual aids.
- eggs = 1223
- tomato = 123412
- sandwich = 12345678
Have you figured it out? If not, here are a few more.
- book = eggs = 1223
- church = tomato = 123412
- breaking = sandwich = 12345678
Hopefully, you figured it out! If not, it’s ok! Take the word book, the first unique letter is “b”, so it gets a 1. The second unique letter when reading from left to right is “o”, so it gets a 2. Now, the third letter is an “o” and since we’ve already assigned it a value, we do nothing. The third unique letter is “k”, so it gets a value of 3. Thus, “book” can be rewritten as “1223”, just the same as “eggs”. Message me if it’s not clear.
This little game is simultaneously a vocabulary builder, as well as a spelling “test”, as well as an introduction to permutations and enumerations.
Suppose I give you a “numerical spelling” like 1223. Can you find a word that fits that pattern? There can be many words! For examples, book, look, took, hook, rook, loot, tool, fool, eggs, eggy, keep, deep, and so forth. But not, begs (that’s 1234) nor peep (that’s 1221).
You can crank up the difficulty by restricting the words to a certain theme. What is a math word that follows the pattern 122? add, odd, as two examples.
As I said, you can do this as an “I spy” activity or as a free form. I have found both to be equally enjoyable. Though, for younger kids, the “I spy” version is more engaging! For older kids and adults, the free form version is just as fun.
Activity #3, A One-Timer from the Twitterverse
If you didn’t participate in this activity on Twitter, now’s your chance!
here's a challenge for you! tweet a #math word to me (lower case) and I'll give it two scores A and B. how i'm scoring it will be a mystery! 🙂 your task is to get a word that has a score for A >= 4 or for B >= 1
hints:
hyperbola —> A = 1, B = 0
statistics —> A = 3, B = 0— M Shah (@shahlock) June 22, 2019
I received a ton of submissions here. And we had a few folks who were able to solve this! Check out their solutions.
@jonathanavt was the first to deduce what generated A and B. His submisson of “hilbert” fits the bill!
Finally found one that satisfies both and is math-related: hilbert (4, 1). At least I didn’t think bottle (4, 1) would count by itself.
— Jonathan👣🚲 (@jonathanavt) June 22, 2019
But he also then realizes …
fifth (4, 1) (high mean)
fifteenth (5, 1)
thirtieth (5, 1)
thirty-fifth (6, 1) (low mean)
fiftieth (5, 1)
fifty-third (5, 1) (low mean)
fifty-fifth (6, 1)
hundredth (5, 1)And so on. I didn’t think to try this supply. I won’t automate the search for our sanity.
— Jonathan👣🚲 (@jonathanavt) June 22, 2019
Next up, @Mathistopheles nailed it with a nice probing of the function. The submission “breadth” meets the minimum requirements for A and B!
OK, so my submission for A>=4, B>=1 is "breadth". Has that been suggested yet?
— Thomas Oléron Evans (@Mathistopheles) June 22, 2019
Check out his inquiry method
ten
net
odd
odds— Thomas Oléron Evans (@Mathistopheles) June 22, 2019
Also, @kayno1 figured it out!
I’m now sure I know condition B can I test the following : trillionth is (5,0.5)
— Saul Kay (@kayno1) June 22, 2019
@srcav got A worked out. But B remained elusive.
Think i have A now. Stillno clue on B! wjat is B for parallel and Parallelpiped?
— Cav (@srcav) June 22, 2019
@SFrancismath also got A with a nice submission of “differential”.
differential, A=5
Still confused by B.— Steven Francis (@SFrancismath) June 22, 2019
Ruh roh …
God damn it you both nerd sniped me and I've been thinking about this for 50 minutes straight. I'm gonna sleep on it because I M U S T F I G U R E I T O U T
— 3D Printyboi ♥️⚔️💙 (@TetrisIsSexy) June 22, 2019
Next we have the ever-creative @icecolbeveridge in the fray.
Weight
Height
Eight
Eighteen
Eighty
Right(No rush 😉 )
— Colin Beveridge (@icecolbeveridge) June 22, 2019
His submission of “billionth” was a winner, even if it was stumbled upon.
Meanie! 😉 Heh, the B was a bonus, I don’t know what’s going on there (yet).
— Colin Beveridge (@icecolbeveridge) June 22, 2019
@sxpmaths sent in the shocker “syzygy” for A = -4, B = -1! I considered it a winner on magnitude!
well that's the most unique one yet and i think you've found an exceptionally fun to boot!
syzygy
A = -4
B = -1I'm going to call this a winner in the other direction!! nicely done.
— M Shah (@shahlock) June 22, 2019
I learned a new word from @math_doc_ron!
widdershins
— ron taylor (@math_doc_ron) June 22, 2019
Follow the original tweet to see even more replies.
You can also take a look at the words submitted and see if you can figure out what the scoring schemes for A and B are. Below this table are the spoiler solutions.
| word | A score | B score | |
|---|---|---|---|
| 0 | add | 2 | 1.0 |
| 1 | added | 3 | 1.0 |
| 2 | addend | 3 | 0.5 |
| 3 | adding | 1 | 0.0 |
| 4 | adds | 2 | 0.5 |
| 5 | altitude | 4 | 0.5 |
| 6 | angle | 0 | 0.0 |
| 7 | antiwiddershins | 4 | 0.0 |
| 8 | arc | 0 | 0.0 |
| 9 | arcs | 0 | 0.0 |
| 10 | billionth | 5 | 1.0 |
| 11 | calculus | 2 | 0.0 |
| 12 | chi | 1 | 0.0 |
| 13 | circle | 1 | 0.0 |
| 14 | circumference | 1 | 0.0 |
| 15 | concatenate | 2 | 0.0 |
| 16 | cone | 0 | 0.0 |
| 17 | counterclockwise | 3 | 0.0 |
| 18 | counterwiddershins | 4 | 0.0 |
| 19 | dart | 2 | 0.5 |
| 20 | delimited | 4 | 0.0 |
| 21 | depth | 2 | 1.0 |
| 22 | derivative | 2 | 0.0 |
| 23 | differential | 5 | 0.0 |
| 24 | eight | 1 | 0.0 |
| 25 | eighteen | 1 | 0.0 |
| 26 | eighty | 0 | 0.0 |
| 27 | eta | 1 | 0.0 |
| 28 | fold | 3 | 1.0 |
| 29 | fractal | 3 | 0.0 |
| 30 | gradient | 1 | 0.0 |
| 31 | hausdorff | 4 | 0.0 |
| 32 | height | 2 | 0.5 |
| 33 | heights | 2 | 0.0 |
| 34 | hilbert | 4 | 1.0 |
| 35 | hubwise | 2 | 0.0 |
| 36 | hundred | 3 | 0.0 |
| 37 | hyperbola | 1 | 0.0 |
| 38 | isoperimetric | 0 | 0.0 |
| 39 | jacobian | 0 | 0.0 |
| 40 | julia | 0 | 0.0 |
| 41 | klein | 2 | 0.0 |
| 42 | latitudinal | 5 | 0.0 |
| 43 | limit | 2 | 0.0 |
| 44 | log | 0 | 0.0 |
| 45 | logarithm | 2 | 0.0 |
| 46 | logs | 0 | 0.0 |
| 47 | longitude | 2 | 0.0 |
| 48 | matrix | 1 | 0.0 |
| 49 | mu | 0 | 0.0 |
| 50 | net | 1 | 0.0 |
| 51 | nets | 1 | 0.0 |
| 52 | node | 1 | 0.0 |
| 53 | nu | 0 | 0.0 |
| 54 | odd | 2 | 1.0 |
| 55 | odds | 2 | 0.5 |
| 56 | one | 0 | 0.0 |
| 57 | packing | -1 | 0.0 |
| 58 | parallelogram | 1 | 0.0 |
| 59 | parameter | 0 | 0.0 |
| 60 | phi | 0 | 0.0 |
| 61 | pi | -1 | -0.5 |
| 62 | pie | -1 | 0.0 |
| 63 | planar | 0 | 0.0 |
| 64 | plot | 1 | 0.5 |
| 65 | plots | 1 | 0.0 |
| 66 | prime | -1 | 0.0 |
| 67 | probabilistically | 4 | 0.0 |
| 68 | project | -1 | 0.0 |
| 69 | proportional | 0 | 0.0 |
| 70 | psi | -1 | 0.0 |
| 71 | quadrilateral | 3 | 0.0 |
| 72 | rho | 1 | 0.0 |
| 73 | rhombicosidodecahedral | 7 | 0.0 |
| 74 | right | 1 | 0.0 |
| 75 | semiperimeter | 0 | 0.0 |
| 76 | sesquilinear | 0 | 0.0 |
| 77 | set | 1 | 0.0 |
| 78 | sets | 1 | 0.0 |
| 79 | sine | 0 | 0.0 |
| 80 | sines | 0 | 0.0 |
| 81 | solution | 2 | 0.0 |
| 82 | solutions | 2 | 0.0 |
| 83 | statistical | 4 | 0.0 |
| 84 | statistically | 4 | 0.0 |
| 85 | statistics | 3 | 0.0 |
| 86 | strength | 2 | 0.0 |
| 87 | subtraction | 3 | 0.0 |
| 88 | sum | 0 | 0.0 |
| 89 | syzygy | -4 | -1.0 |
| 90 | tan | 1 | 0.0 |
| 91 | tangent | 1 | 0.0 |
| 92 | tau | 1 | 0.0 |
| 93 | ten | 1 | 0.0 |
| 94 | tend | 2 | 0.5 |
| 95 | tens | 1 | 0.0 |
| 96 | tetrahedron | 4 | 0.0 |
| 97 | third | 3 | 1.0 |
| 98 | three | 2 | 0.0 |
| 99 | three | 2 | 0.0 |
| 100 | ton | 1 | 0.0 |
| 101 | tons | 1 | 0.0 |
| 102 | topology | -1 | 0.0 |
| 103 | tree | 1 | 0.0 |
| 104 | trees | 1 | 0.0 |
| 105 | two | 1 | 0.0 |
| 106 | vector | 1 | 0.0 |
| 107 | weight | 1 | 0.0 |
| 108 | widdershins | 3 | 0.0 |
| 109 | xi | 0 | 0.0 |
| 110 | zero | 0 | 0.0 |
Solving for A and B
If you probed around enough and recognized that B never seems to exceed 1 in magnitude and only appears to take on values of -1, -0.5, 0, 0.5, and 1, maybe you came to the conclusion that it must be some sort of median. Odds are, though, you needed to figure out A first. A it turns out is a “net height” measurement.
The letters “aceimnorsuvwxz” each have a height of 0, “bdfhklt” have a height of 1, and “gjpqy” are below sea level with a “height” of -1. So, the word “probability” has a net height of \(-1 + 0 + 0 + 1 + 0 + 1 + 0 + 1 + 0 + 1 + -1 = 2\). This is the calculation for A.
Since each letter maps to -1, 0, or 1, then each word is a collection of these numbers. The median of these numbers is B!
This is a great little math-word puzzle, but best suited for older travelers with some patience. You can also use this as a classroom exercise perhaps in a stats lesson or for your AP Stats or Calculus class! It’s a great vocab builder!
Finally, here’s a little data viz for you of all words in ENABLE
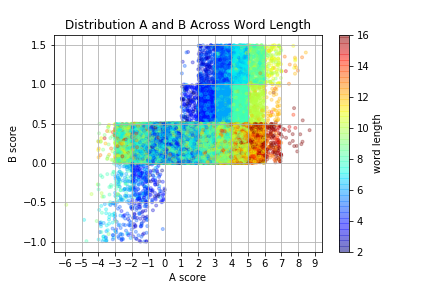
Distribution of A and B with word length as a color overlay. Any point in a “box” has A score equal to the left boundary of the box and B score equal to the bottom boundary of the box.
Hope you enjoyed this! Happy excursioning!